• Home
• News
• Research
• Publications
• People
• Teaching
• Scripts
• Jobs
• Links
• Contact

---

Other Groups at ITP

University > Physics and Astronomy > ITP> Groups

English

Introduction

Amphiphilic molecules have both polar and non-polar parts (eg a polar headgroup connected to a hydrocarbon chain). Important examples are tensides and lipids. We speak of binary amphiphilic systems if the amphiphile is mixed with a polar or a non-polar solvent like water or oil; and of ternary amphiphilic systems if the amphiphile is mixed with both a polar and a non-polar solvent. Due to the hydrophobic effect, the amphiphiles self-assemble into aggregates. In binary amphiphilic systems the basic aggregates are (spherical or cylindrical) micelles and bilayers; in ternary amphiphilic systems, monolayers form at the oil-water interfaces. Depending on temperature, concentration and salt content, these aggregates form many different phases each of which corresponds to a specific arrangement of the amphiphilic aggregates' neutral surfaces. One of the intriguing aspects of amphiphilic polymorphism is the existence of bicontinuous phases which can be traversed in any direction in both the hydrophilic (water-like) and the hydophobic (oil-like) regions. This bicontinuity has been demonstrated by measuring diffusion properties with nuclear magnetic resonance. In contrast to sponge and microemulsion phases, which are bicontinuous as well, bicontinuous cubic phases show long range order which can be demonstrated by the appearance of Bragg-peaks in diffraction patterns. Although bicontinuous ordered phases could have any of the 230 three-dimensional space groups, experiments show that most of them have one of the 36 cubic ones. The amphiphilic interfaces of bicontinuous cubic phases form triply periodic surfaces (TPS). Triply periodic means having a three-dimensional Bravais lattice. It is easy to see that two- or one-dimensional Bravais lattices (like with the doubly periodic hexagonal phase or the singly periodic lamellar phase, respectively) cannot correspond to structures which are continuous both in their hydrophilic and hydrophobic regions.

A TPS divides space into two unconnected but intertwined labyrinths. Both labyrinths percolate space and provide the pathways which can be used to traverse the structure both in the hydrophilic and the hydrophobic regions. In most relevant cases, the two labyrinths are congruent to each other. Then the structure is called balanced. A balanced structure is characterized by two (rather than by one) space groups: the space group H of a single labyrinths and the space group G of both labyrinths together. H can be also be considered to be the space group of the oriented TPS as opposed to G, the space group of the unoriented TPS.  H is a subgroup of index 2 of G since it comprises all symmetry operations of G expect the one operation alpha which interchanges the two labyrinths (i.e. maps one side of the TPS onto the other side). G is a supergroup of H since it comprises all symmetry operations of H plus alpha. Therefore G = H x Z_2 with Z_2 = {1, alpha} being the cyclic group of order 2 which has as its members the unit operation and alpha.

Ternary amphiphilic systems

In ternary amphiphilic systems with water, oil and amphiphile, water and oil regions are separated by amphiphilic monolayers and bicontinuous cubic phases can come in three structural types:

• Single structure: there is only one TPS covered by an amphiphilic monolayer and the two labyrinths are filled with water and oil, respectively.
• Double structure of typ I: the two labyrinths of the single structure are filled with oil. Thus the TPS of the single structure is covered by a water-filled (reversed) bilayer, i.e. a sheet-like water region enclosed by two amphiphilic monolayers.
• Double structures of typ II: this is the case before with water and oil interchanged. Thus the TPS of the single structure is covered by an oil-filled (normal) bilayer, i.e. a sheet-like oil region enclosed by two amphiphilic monolayers.

Since one picture says more than thousands words, look at the following picture which depicts the essential elements of the so-called P-structures:

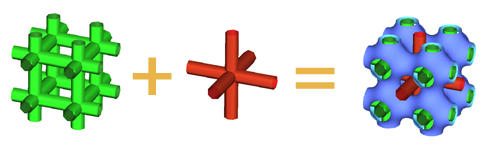
On the left you see the two labyrinths in green and red, respectively. They are congruent to another, and the operation which maps one onto the other is a translation by half the unit cell's body diagonal. In each vertex, six channels meet each other perpendicularly. Each labyrinth has the space group H = Pm3m (simple cubic). If we now plug one labyrinth into the other and identify green and red, we get the new structure on the right which has space group G = Im3m (body centered cubic).  The dividing surface is triply periodic and shown in blue. Note that by construction, the translation by half the unit cell's body diagonal not only interchanges the two labyrinths, but also appears to map the TPS onto itself. Thus the surface has space group G = Im3m also. However, this is not totally correct, since at the same time the two sides of the surfaces are interchanged. As explained above, this means that only the unoriented surface has space group G = Im3m; if we consider the TPS to have two sides (e.g. light and dark blue or green and red or white and black), this operation is not a symmetry operation and the space group is H = Pm3m only. (In fact, this kind of additional symmetry is called a color symmetry and constructions like this are also known from other part of condensed matter physics like magnetic crystals, Fermi surfaces and zero potential surfaces in ionic crystals). Now how do the P-structures look like in a physical system like the ternary amphiphilic system ? For the single P, the green labyrinth might be filled with oil and the red one with water. The blue surface is an amphiphilic monolayer. For double structures of typ I and II both labyrinth are filled with the same component, i.e. oil and water, respectively. Then the TPS is covered by two monolayers which sandwich the water and oil region, respectively.

Binary amphiphilic systems

If we remove the oil, the ternary system becomes a binary amphiphlic system with water and amphiphile. However, there are still three different structural types possible:

• Single structure: one network of cylindrical micelles with space group H in a water matrix.
• Double structure of typ I: two networks of cylindrical micelles with space group G in a water matrix.
• Double structures of typ II: two networks of water channels with space group G separated by an amphiphilic bilayer. Or expressed differently: two networks of inverse cylindrical micelles filled with water (thus these phases are often called inverse cubic phases).

Due to their biological relevance, inverse cubic phases are well investigated with lipid-water mixtures (work by Luzzati, Fontell, Seddon, Templer, Hyde, Anderson, etc). The most common one corresponds to the G-surface, but D- and P-surfaces are established also. The sponge phase can be considered to be a molten variant of the inverse cubic phases, although of a lattice constant much larger than one normally finds for inverse cubic phases. For binary double structures of typ I, only the G-surface is confirmed (for surfactant-water systems). I do not know any experimental account of binary single structures. For ternary systems, much less experimental results are known. One exception is the system DDAB-water-hydrophobe, which was investigated by several authors. Stroem and Anderson report that here five different cubic phases exist, all of them being ternary double structures of typ II. Although many ternary amphiphilic systems feature cubic bicontinuous phases, indexing seems to be much more difficult here than with binary amphiphilic systems. Since the ubiquitous microemulsion consists of amphiphilic monolayers separating continuous regions of water and oil, one expects to find ternary single structures also. However, except for one or two papers (e.g. by Raedler et al.), not much has been reported by experimentalists along these lines. On the other hand, in the Ginzburg-Landau models I worked with, the simple structures seem to be quite favorable both for binary and ternary systems. A stable single structure was also found for a lattice gas model for binary systems by Linhananta and Sullivan. Therefore it well might be that the experimentalists have not looked closely enough yet. This stands in marked contrast to the fact that there is a very nice experimental way to get a characteristic signature of balanced single structures: by deuterating the components, one can switch from bulk to film contrast and thus from measuring spacegroup H to measuring spacegroup G in small angle neutron scattering (doing the same for a double structure should not yield any change in space group).

Bicontinuous cubic phases based on triply periodic minimal surfaces

Click here for a description of our own research, related links and selected references.

Last modified Mon Nov 13 10:05:20 MET 2000.
Back to home page Ulrich Schwarz.