|
|
Mechanics and regulation of the actin cytoskeleton
|
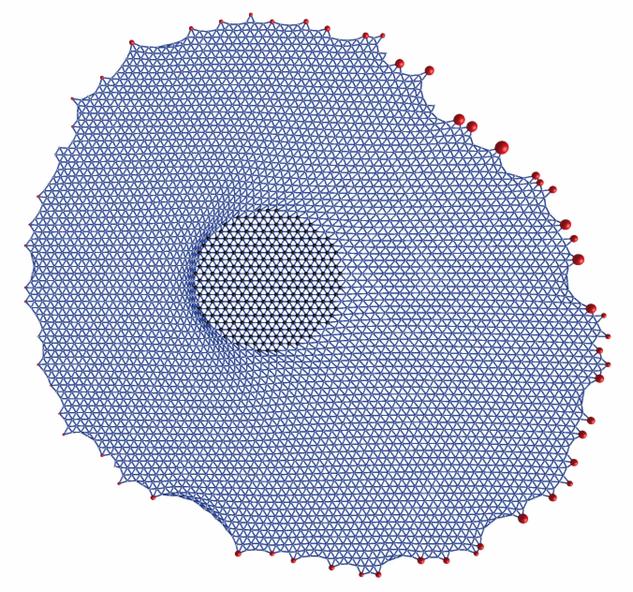
| Model for stress propagation in a pre-stressed and homogeneous actin cytoskeleton: force is applied to the left in the circular domain in the middle and propagated to the focal adhesions whose positions are taken from a real experiment. One sees that force (represented by the sizes of the red dots) is relaxed at the left and increased at the right. |
The actin cytoskeleton is the main determinant of the mechanics of mammalian cells. In general, it is very dynamic and exists in many different morphologies, which are determined by a large variety of internal and external signals. Modelling the actin cytoskeleton becomes feasible if one focuses an a special situation of interest. Recently we have approached this challenge for two such special cases: for a homogeneous network spanning the cell body between discrete sites of adhesion and for stress fibers, which are thick and contractile actin bundles.
For the homogeneous case, we have used the concept of cable networks reflecting the polymer nature of the actin filaments (they buckle under compression but tense as springs under tension). Using two different variants of this approach, we have studied the propagation of external force through the actin network towards focal adhesions (Paul et al., Biophysical Journal 2008, passive cable networks) and the shape of cells on micropatterned substrates (Bischofs et al., Biophysical Journal 2008, actively contracting cable networks).
For stress fibers, we have developed a one-dimensional viscoelastic model which in the continuum limit can be formulated as a partial differential equation for the displacement field. Recently we have used this stress fiber equation to analyze the experimental results of laser cutting experiments (Colombelli et al., Journal of Cell Science 2009; Besser et al., Physical Review E 2011). Moreover we have formulated for the first time a model for the main signal transduction pathway regulating myosin contraction along stress fibers. Our model for the Rho-pathway is a system of reaction-diffusion equations. Because the Rho-signal is triggered by force at focal adhesions which in turn is created by the Rho-mediated contractility in the stress fibers, we arrived at a system of equations which couple biochemical and mechanical aspects of cell adhesion in a positive feedback loop (Besser and Schwarz, New Journal of Physics 2007 and Biophysical Journal 2010).
Publications:
- R. Paul, P. Heil, J. P. Spatz and U. S. Schwarz. Propagation of mechanical stress through the actin cytoskeleton towards focal adhesions: model and experiment. Biophys. J., 94:1470-1482, 2008. (abstract, doi:10.1529/biophysj.107.108688, PDF)
- I. B. Bischofs, F. Klein, D. Lehnert, M. Bastmeyer, and U. S. Schwarz. Filamentous network mechanics and active contractility determine cell and tissue shape. Biophys. J., 95:3488-3496, 2008. (abstract, PDF)
- J. Colombelli, A. Besser, H. Kress, E.G. Reynaud, P. Girard, E. Caussinus, U. Haselmann, J.V. Small, U. S. Schwarz, and E.H.K. Stelzer. Mechanosensing in actin stress fibers revealed by a close correlation between force and protein localization. J. Cell Sci., 2009. (abstract, doi: 10.1242/jcs.042986, PDF)
- Achim Besser, Julien Colombelli, Ernst H. K. Stelzer, and Ulrich S. Schwarz. Viscoelastic response of contractile filament bundles. Phys. Rev. E, 83(5):051902, May 2011. (abstract, doi:10.1103/PhysRevE.83.051902, qbio arXiv 1102.5295, PDF, Supplemental material)
- A. Besser and U. S. Schwarz. Coupling biochemistry and mechanics in cell adhesion: a model for inhomogeneous stress fiber contraction. New J. Phys., 9:425, 2007. (abstract, qbio arXiv:0707.2551v2, doi:10.1088/1367-2630/9/11/425, PDF)
- A. Besser and U. S. Schwarz. Hysteresis in the cell response to time-dependent substrate stiffness. Biophys. J., 99:L10-L12, 2010. (abstract, doi:10.1016/j.bpj.2010.04.008, qbio arXiv 1007.1092, PDF, Supplemental material)
Last modified Di 4. Okt 17:45:17 CEST 2011
by USS.
Back to home page Ulrich Schwarz.